Technical understanding part 1

Considerations made about the structural integrity of a design solution

6.1b Show an understanding of the processes that can be used to ensure the structural integrity of a product, such as:

• Triangulation

A triangle is one of the strongest shapes available for engineers to use. It is used to create a shape that is rigid and will not move. We find these shapes regularly used in the building industry to keep building upright and rigid.

Click on this link for more information on triangulation.

• Reinforcing

Reinforcing is used to strengthen materials and improve either their compressive or tensile strength.

How mechanisms provide functionality to products and systems.

6.2a Demonstrate an understanding of the functions that mechanical devices offer to products, providing different types of motion, including:

• rotary

• linear

• reciprocating

• oscillating.

Below is a Peg and Slot mechanism, this converts rotary to oscillating motion. However, the output is reciprocating motion.

• To learn about converting from one type of motion to another, click here for linkages and here for rotary motion.
• You can learn more about gear trains here.
• You can learn about pulley systems here.

Sample Mechanisms from exam paper:

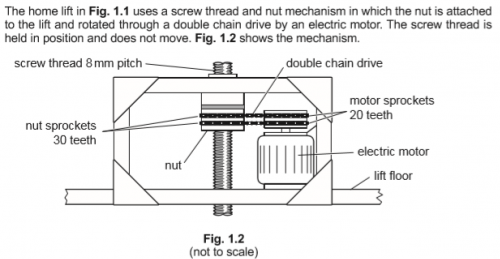
i. The lift moves at a speed of 0.08 m s–1.

   Calculate the time taken in seconds (s) for the lift to rise between floors which are 2800 mm apart. Show your working.

   Speed = distance/time Time = distance/speed (1). 
   Conversion of 2800 mm to 2.8 m (1). 
   Time = 2.8/0.08 Time = 35 s (1).

ii. Analysing the data in Fig. 1.2, calculate the motor rotational speed required in revolutions per minute (rpm) to cause the nut to climb up the thread at a speed of 0.08 m s–1.

   Show your working.

 In 1 second, number of nut revolutions = linear distance / thread pitch = 80/8 = 10 revolutions (1). 
 Sprocket gear ratio = driven / driver = 30/20  Motor revolutions in 1 second = 10* x 30/20 = 15 revolutions (1). 

OR

 Calculate that the nut rotation at 10 revolutions is 600rpm therefore at 15 revolutions multiply by 1.5 = 900rpm (1) 
 Conversion to rpm: Motor rotational speed = 15*x60 = 900 rpm (1).

iii. Give two reasons why a double chain drive is used in this application.

• Safety – if one chain fails there is a backup (1).
• The two chains share the load (1).
• Each chain can be thinner which could save cost, reduce weight, and allow a more compact drive system (1).
• If one chain fails, the lift will continue to work (1).
• They are more secure in instances where a belt drive might be used due to them being non slip drives (1).
• Any other valid suggestion.

iv. The maximum total mass of the lift and occupants is 350 kg.

- Calculate the power required in watts (W) to raise the 350 kg lift at a velocity of 0.08 m s–1.

- Show your working.

- gravitational potential energy = mgh

- power = E/t

- gravitational field strength, g = 9.81 N kg–1

   Showing understanding that the lift rises 0.08m in 1s (1). i.e. 0.08 = h (1) 
   GPE = mgh = 350x9.81x0.08 = 274.68 J (1).  
   Showing understanding that the power required is equal to the increase in GPE in 1s (or for ‘calculating’ the power): 
   Power = E/t = 274.68*/1 =      274.68 W (1). I.e. time = 1s

6.2b Demonstrate an understanding of devices and systems that are used to change the magnitude and direction of forces and torques, including:

1. For gears, cams, pulleys and belts, levers, linkages, screw threads, worm drives, chain drives and belt drives, click on this here to see images and explanations of gears, cams, pulleys, levers, chain drives and belt drives.
2. Screw threads and worm drives are often used to control linear motion in CNC machines, such as 3D printers.

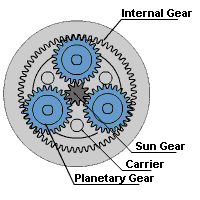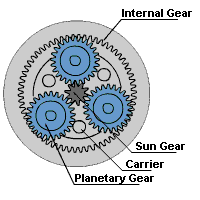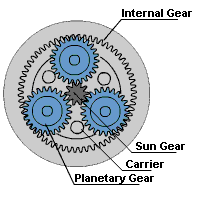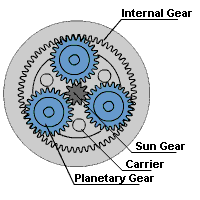
Epicyclic gear systems

1. An epicyclic gear train consists of two gears mounted so that the center of one gear revolves around the center of the other. A carrier connects the centers of the two gears and rotates to carry one gear, called the planet gear, around the other, called the sun gear. The planet and sun gears mesh so that their pitch circles roll without slip. A point on the pitch circle of the planet gear traces an epicycloid curve. In the simplified case in the image below, the sun gear is fixed and the planetary gear(s) roll around the sun gear.

1. An epicyclic gearbox has a co-linear (in-line) input and output shaft which enables the design of a product to be more compact.
2. Epicyclic gears can handle higher torque than a compound gear chain because of their design, which is required in products like food mixers and drills.
3. Epicyclic gearbox is compact which leads to a smaller product.

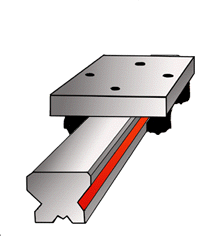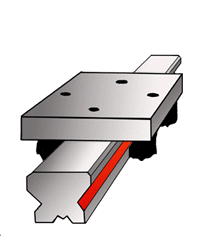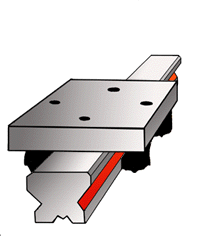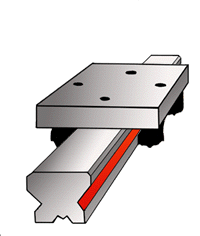
Bearings

• See textbook, pg. 251 for more.
• A bearing is a component which supports a moving part and allows it to move only in the desired motion, with little friction. Bearings are most commonly used to support a drive shaft so it can rotate freely. A drive shaft needs to

be supported at a minimum of two points along its length (sometimes more in high-load applications) so that the shaft is held accurately in place, ensuring that gears and other components stay in precise mesh with each other. The forces acting on a shaft can be radial forces which try to push the shaft sideways, or axial forces which try to push the shaft along its axis.

• A bearing is a machine element that constrains relative motion to only the desired motion, and reduces friction between moving parts. The design of the bearing may, for example, provide for free linear movement of the moving part or for free rotation around a fixed axis; or, it may prevent a motion by controlling the vectors of normal forces that bear on the moving parts. Most bearings facilitate the desired motion by minimizing friction. Bearings are classified broadly according to the type of operation, the motions allowed, or to the directions of the loads (forces) applied to the parts.
• Using bearings in the rotating parts of the mechanical systems (e.g. on a car park barrier gate) reduces the torque required to overcome frictional losses and increases the mechanical efficiency of the system.
• Reducing static friction in systems enables parts to start moving quicker meaning they can operate more rapidly, allowing increased productivity.
• Bearings reduce wear between mechanical parts, increasing the longevity / life of the mechanical system.
• Reducing friction will also reduce the noise created, which may be desirable in a commercial product.

Lubrication

• A lubricant is a substance, usually organic, introduced to reduce friction between surfaces in mutual contact, which ultimately reduces the heat generated when the surfaces move. It may also have the function of transmitting forces, transporting foreign particles, or heating or cooling the surfaces. The property of reducing friction is known as lubricity.
• Lubrication can also protect ferrous metal parts from corrosion, as the oil/grease will prevent air and moisture from being able to attack the metal.
• Where moving parts are under-lubricated or not lubricated, friction between these surfaces will lead to an increase in temperature at the joint / system. Over time, this can result in parts fusing/welding themselves together, leading to system failure. May also lead to...
  • Increased wear and tear of joint / system resulting in quickened fatigue and failure of joint / system.
  • Parts escaping intended joint / system causing issues and damaging others system, e.g. electronics.
  • Slippage of joint / system leading to inefficient performance of joint / system.
  • Difficulties for future maintenance causing increases to maintenance costs and maintenance time required.
• Types of lubricants:
  • Mineral Oil
  • Synthetic oils
  • Solid Lubricants
  • Aqueous Lubricants
  • Bio Lubricants

Efficiency in mechanical systems

1. Mechanical efficiency measures the effectiveness of a machine in transforming the energy and power that is input to the device into an output force and movement. Efficiency is measured as a ratio of the measured performance to the performance of an ideal machine:

  Efficiency = Measured performance/Ideal performance
  or
  Efficiency = (Mechanical Advantage X 100)/Velocity Ratio

2. Because the power transmission system or mechanism does not generate power, its ideal performance occurs when the output power equals the input power, that is, when there are no losses. Real devices dissipate power through friction, part deformation and wear.

3. The ideal transmission or mechanism has an efficiency of 100%, because there is no power loss. Real devices will have efficiency less than 100% because rigid and friction-less systems do not exist. The power losses in a transmission or mechanism are eventually dissipated as heat.

The forces that need consideration to ensure structural and mechanical efficiency.

6.3a Demonstrate an understanding of static and dynamic forces in structures and how to achieve rigidity, including:

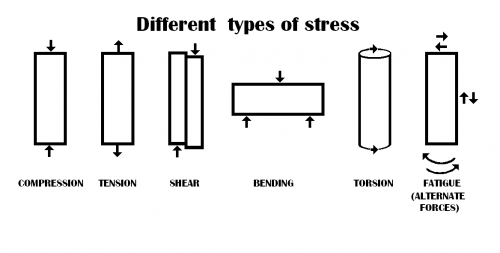
• Tension, compression, torsion and bending.

1. Tension may describe the pulling force transmitted axially by means of a string, cable, chain, or similar one-dimensional continuous object, or by each end of a rod, truss member, or similar three-dimensional object; tension might also be described as the action-reaction pair of forces acting at each end of said elements. Tension could be the opposite of compression.

2. Compression is the application of balanced inward ("pushing") forces to different points on a material or structure, that is, forces with no net sum or torque directed so as to reduce its size in one or more directions.

3. Torsion is the twisting of an object due to an applied torque. Torsion is expressed in newton per squared meter (Pa) or pound per squared inch (psi) while torque is expressed in newton metres (N·m) or foot-pound force (ft·lbf).

4. Bending (also known as flexure) characterises the behaviour of a slender structural element subjected to an external load applied perpendicularly to a longitudinal axis of the element.

• Stress, strain and elasticity.

1. Stress is a physical quantity that expresses the internal forces that neighboring particles of a continuous material exert on each other.

2. Strain is the measure of the deformation of the material. For example, when a solid vertical bar is supporting a weight, each particle in the bar pushes on the particles immediately below it. When a liquid is in a closed container under pressure, each particle gets pushed against by all the surrounding particles.

3. Elasticity is the ability of a body to resist a distorting influence or deforming force and to return to its original size and shape when that influence or force is removed.

• Mass and weight.

1. Mass is both a property of a physical body and a measure of its resistance to acceleration (a change in its state of motion) when a net force is applied.[1] It also determines the strength of its mutual gravitational attraction to other bodies. The basic SI unit of mass is the kilogram (kg).

2. Weight of an object is usually taken to be the force on the object due to gravity.[1][2] Weight is a vector whose magnitude (a scalar quantity), often denoted by an italic letter W, is the product of the mass m of the object and the magnitude of the local gravitational acceleration g;[3] thus: W = mg. The unit of measurement for weight is that of force, which in the International System of Units (SI) is the newton.

• Rigidity.

1. Rigidity is the property of a solid body to resist deformation. Structural rigidity, a mathematical theory of the stiffness of ensembles of rigid objects connected by hinges.

• Modes of failure.

1. Mechanical failure. Some types of mechanical failure mechanisms are: excessive deflection, buckling, ductile fracture, brittle fracture, impact, creep, relaxation, thermal shock, wear, corrosion, stress corrosion cracking, and various types of fatigue.

Design Engineering homepage .